 Plate
Driving Forces and Tectonic Stress
Plate
Driving Forces and Tectonic StressStructure Seminar
Arlo Brandon Weil
 Plate
Driving Forces and Tectonic Stress
Plate
Driving Forces and Tectonic Stress
Structure Seminar
Arlo Brandon Weil
University of Michigan, Ann Arbor, MI
click here for
spinning globe
Table of Contents
INTRODUCTION
"In science a phenomenon or a hypothesis can become so familiar
and its utility in providing as explanation, or consistent description
of a greater number of diverse facts so evident, that the underlying mechanism
may often be left unstudied"
(Runcorn, 1980)
![]()
WHAT Drives Plate Tectonics ???? This question has been the subject of intense debate ever since the plate tectonic theory was first eccepted by the geologic community in the late 1960's. The major concern is whether mantle convection and the activity of mantle plumes dominate the driving forces of plate motion, or whether surface boundary and plate forces, such as slab pull and ridge push provide the most important forces. The argument is basically whether the plates are passively riding along on the top of a mantle convection cell, or whether the plates themselves the active drivers, dragging along with them the mantle below.
To begin understanding and evaluating the different forces involved in the plate tectonic process, we must first isolate these forces and define their physical and mechanical properties. Once we have done that, we must make sure that any hypothesis or model that we devise to produce these forces is compatible with the observations and characteristics that we know and understand about the Earth. Mainly, is the model (1) compatible with the rigid behavior of lithospheric plates, (2) compatible with the wide variety of plate sizes, geometry, type, and motion; does it (3) satisfy the existence of complex plate boundary conditions, (4) provide enough energy to account for all the motion; is it able to (5) produce the observed tectonic stresses observed in the upper lithosphere; and does it (6) satisfy the long-lived steady state relative plate motions (on the order of tens of millions of years), as well as sudden dramatic changes in motion we observe from modeled plate reconstructions (i.e., the Pacific plate circa 43 Ma).
With this basic set of plate driving force parameters and conditions developed, we can try to relate our predicted forces back to the causal effects of tectonics at the Earth's surface. To date, our best tool for observing the effects of plate driving forces (PDF's) is the existence of large-scale tectonic stresses. Tectonic stresses result from plate driving forces. Therefore, using measured data for the Earth's lithosphere, we can begin to think quantitatively about the different magnitudes of the involved forces.
There are several methods one can use to quantify PDF's, namely: (1) finite element deformation modeling, using the inter-plate stress fields to constrain the driving forces, (2) empirical mathematical relationships between plate boundaries, plate age, type, and velocity, and (3) active Net Torque analysis. Compared to the others, the last method does a better job at accounting for all the large number of active forces as well as the complex boundary conditions and plate characteristics that our PDF model must satisfy.
Using a combination of the known tectonic stresses along with a quantified
force relationship, we should be able to devise an accurate account of
all the active forces involved in today's plate motion. In addition, we
should better understand the magnitude scale for the different categories
of mantle and plate forces.
![]() The
Active Forces Involved in the Driving of Lithospheric Plates
The
Active Forces Involved in the Driving of Lithospheric Plates
As stated earlier, in order to fully understand
what drives the lithospheric plates of the Earth, we must first identify
and understand the forces involved. A number of forces have been postulated
since the dawn of the tectonic theory, including ridge push, slab pull,
trench suction, collisional resistance, and basal drag (Forsyth et al.,
1975; Richardson, 1992). In the past ten years, many scientists have begun
to assume that the boundary and body forces of the plates, rather than
the frictional drag produced by mantle convection, are the most dominant
group of forces driving plate motions. In the following section, the basic
physical properties of each of the main forces believed to be involved
in the total net motion of plates will be described and defined (fig.1).

Figure 1: Basic schematic of different Plate Driving Forces.
Ridge Push (fig. 2) has been considered in two
different manners, as a body force and as a boundary force. As a body force,
ridge push has been attributed to the cooling and thickening of
the oceanic lithosphere with age (McKenzie, 1968; McKenzie, 1969; Richards,
1992; Vigny et al., 1992). This type of force can be thought of as created
by the horizontal pressure gradient attributable to the cooling and thickening
of the oceanic lithosphere, and calculated as this force integrated over
the area of the oceanic portion of a given plate (Lister, 1975). In this
respect, Ridge Push can be considered a body force, rather than a boundary
force acting over the oceanic part of a plate (Wilson, 1993). When making
such a calculation however, one must take into account that oceanic lithosphere
older than 90 Ma is no longer cooling significantly, and therefore not
contributing to the effective ridge push force (Ziegler, 1993). The alternative,
Ridge Push as a boundary force, is caused by the "gravity
wedging" effect ( Bott, 1993). This effect results from warm, buoyant
mantle upwelling beneath the ridge crest which causes a topography-induced
horizontal pressure gradient. Here the force would be acting as a boundary
force at the edge of the lithospheric plate, proportional to the length
of the ridge, and not as a body force over the entire oceanic portion of
the plate. In both of the above cases Ridge Push would be amplified, by
as much as a factor of two when hot spot activity is centered on a spreading
ridge axis (Ziegler, 1993). This is important when considering the effects
of ridge push as a cumulative force acting on all the plates, and must
be taken into account in any net force calculations.
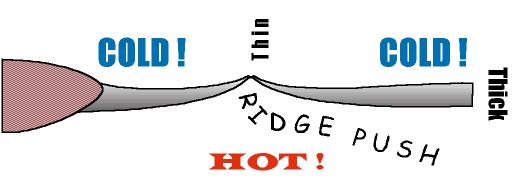
Figure 2: Schematic of Ridge Push forces.
The Slab Pull (fig. 3) forces are derived from the negative
buoyancy of the cold subducting lithosphere and are dependent on the angle,
temperature, age and volume of the subducting slab, as well as the length
of the respective trench (Chapple and Tullis, 1977). Slab Pull is considered
a boundary force, and from most estimates is responsible for some of the
largest forces, or torques in the driving system (Wilson, 1993). Several
empirical studies have shown a strong correlation between plate velocities
and age of subducting oceanic lithosphere for plates with long subduction
boundaries (Forsyth and Uyeda, 1975; Chapple and Tullis 1977). This might
suggest that slab pull is the dominant acting force. However, there are
several plates that have little or no portion of their boundaries subducting
and it is therefore important to look for other contributing forces.
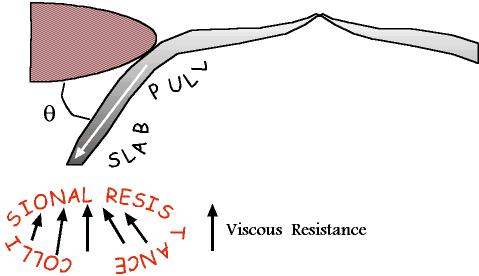
Figure 3 : Schematic of Slab Pull and Collisional Resistance forces.
Related very closely to Slab Pull is Collisional Resistance (fig. 3). For every subducting slab there is an associated resistive force provided by the relatively high viscosity of the warmer, more ductile upper mantle. Together, the negative buoyancy of the sinking slab and the resistive nature of the slab entering the mantle is called the Net Slab Force. The sum of these two forces is exerted at the colliding margin (Ziegler, 1992) and contributes to the intra-plate stress field of the surface plates (Wilson, 1993). Alternatively, recent work has shown that the slab forces may be largely balanced within the slab itself and contribute relatively little to the deformation of the surface plates (Richards, 1992).
Trench Suction (fig. 4) forces are observed in the overriding
plate at subduction zones as a net trenchward pull, often times resulting
in back arc extension (Forsyth and Uyeda, 1975; Chase, 1978). Trench Suction
is thought to result from small-scale convection in the mantle wedge, driven
by the subducting lithosphere. This force is difficult to isolate from
other forces because of how little we know about mantle convection in the
shallow subsurface (Ziegler, 1993). Related to Trench Suction is Slab
Roll-Back. This is caused by the small-scale convection current on
the back-side of subducting slabs. We see this phenomena today in the Hellenic
Arc of Greece, and possibly in the western Pacific. This current produces
a pull away from the trench, consequently rolling back the hinge of the
subducting slab. Both trench suction forces can be thought of as a conservation
of matter argument requiring an asthenospheric counter-current in the wedge-shaped
region between the down-going slab and the upper plate. It is this counter-current
that will result in the trenchward pull of the overriding plate (Chapple
and Tullis, 1977).
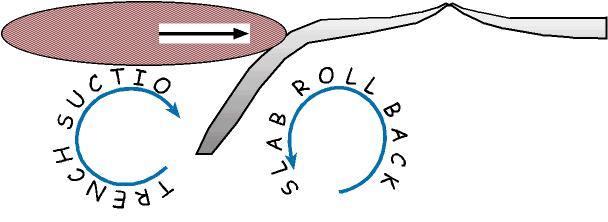
Figure 4: Schematic of Trench Suction forces.
Plate Tectonic Resistive forces (fig.5) are exerted on the overriding plate in a subduction zone at the contact with the descending slab. This force is thought to result in a shear stress that is distributed over the subduction thrust interface, that dips in the direction of the plate's interior (Wilson, 1993). However, tectonic resistive forces are considered equal and opposite in sign to the force exerted on the subducting plate, and therefore do not contribute greatly to the net driving force for plate motion (Meijer and Wortel, 1992).
The last major force, Basal Shear Traction or Basal Drag
(fig. 5) is important because of its relevance to the fundamental question
of whether plate motions are active or passive. Basal Shear Traction
is the resistance or dragging force associated with the interface between
the upper mantle and the lithosphere. Today this force is thought to be
small, but until we know more about the coupling between the lithosphere
and the mantle is better constrained, we cannot be certain how important
it is. It is thought to have a small magnitude per unit area, but when
spread over the entire under-surface of big plates can result in a large
cumulative resistance.

Figure 5 : Schematic of Plate Tectonic ans Basal Shear Traction Resistive
forces.
The lack of good correlation between plate velocity and surface area
has traditionally been used to argue against Basal Shear Traction (BST)
as an important driving force. In recent models researchers have considered
BST a passive force, either driving or resisting plate motion, but not
dominating plate motion (Richardson, 1992). The contribution of BST on
the motion of plates depends on whether the flow pattern at the lithosphere-mantle
interface is radial or unidirectional and parallel or anti-parallel with
respect to the overlying plate motions (Forsyth and Uyeda, 1975; Doglioni,
1990). However, the mechanical nature of this interface and its flow pattern
are unknown. Other researchers are advocates of drag forces playing
an important role in driving plate motion, while the plates remain passive
(Vlaar et al., 1976; Jacoby et al., 1980). In this case the lateral motion
of the plates would be caused by the mantle's exertion of a drag force
on the overriding lithosphere, above warm upwellings, which would subsequently
create a deviatoric stress regime. Here, the Shear Traction is estimated
to be small per unit area and would be proportional to the horizontal,
or toroidal, component of the upper-mantle's flow velocity relative to
the overlying plate velocity (Ziegler, 1993). But it is important to point
out that the mechanics of upper-mantle flow are poorly constrained at this
time.
![]() Tectonic
Stresses and Their Relationship to Plate Driving Forces
Tectonic
Stresses and Their Relationship to Plate Driving Forces
We must now seek out information on the subsequent effects, or in geologic terms, the associated stress related to these forces. In order to do this it is very important that we understand the regional patterns of the present-day tectonic stress field. Tectonic stresses are those stresses produced by the forces that drive plate tectonics (Middleton et al., 1996). Because of their integral relationship to the present motion of plates, the magnitude and direction of tectonic stress is very difficult to predict unless we can measure recent tectonic movement or seismic activity. In order to distinguish a measured tectonic stress from those stress fields that are locally derived, we must look at the spatial uniformity of the in situ stress field. For tectonic stresses the stress fields are typically uniform over distances many times (2 to more than 100 times) the thickness of the elastic part of the lithosphere, while local stresses are only a fraction of that same thickness (Zoback et al., 1989). It is also found that for tectonic fields the three principal stresses lie in approximately horizontal and vertical planes, with the horizontal stress component almost always larger than the vertical component. As a consequence the orientation of the principal stress axes of the measured stress tensor can be constrained by specifying the direction of just one of the horizontal principle stresses (Zoback, 1989). This is convenient for recording and measuring crustal stresses.
Once measured, tectonic stresses can give valuable information about the forces acting on the plates and therefore the dynamics of plate tectonics. A group of some 30 scientists from all over the world, headed by Mary Lou Zoback, have created a working database of in situ stress measurements for most of the Earth's lithospheric plates. They collected over 7300 in situ stress measurements, of which 4400 are considered tectonic stresses. These measurements were taken from bore-hole breakouts, hydraulic fractures, style of active faulting, volcanic alignment, seismic focal mechanisms, and transform fault azimuths. The entire database then underwent a scrutinous quality rating to asses the reliability of the individual data, with any unsatisfactory data discarded (Zoback, 1989; Zoback, 1992).
The World Stress Map Project (WSMP), because of its huge database, has provided significant advancement in our efforts to determine he relative importance of different plate driving forces (fig. 6). The project has also provided constraints on the magnitude of both broad scale and local stresses acting on the lithosphere. Subsequent analysis has shown that a majority of the data can be adequately explained by the geometry of plate boundaries and the conventional ridge push, slab pull, and subduction forces, and do not necessarily require a significant contribution from sublithospheric mantle flow inferred from seismic tomography (Zoback and Magee, 1991; Wilson, 1993). It appears that regionally uniform horizontal intra-plate stress orientations are consistent with either relative or absolute plate motions indicating that plate-boundary and body forces must be the dominant contributors to the stress distribution within plates (Zoback, 1989; Zoback, 1992).
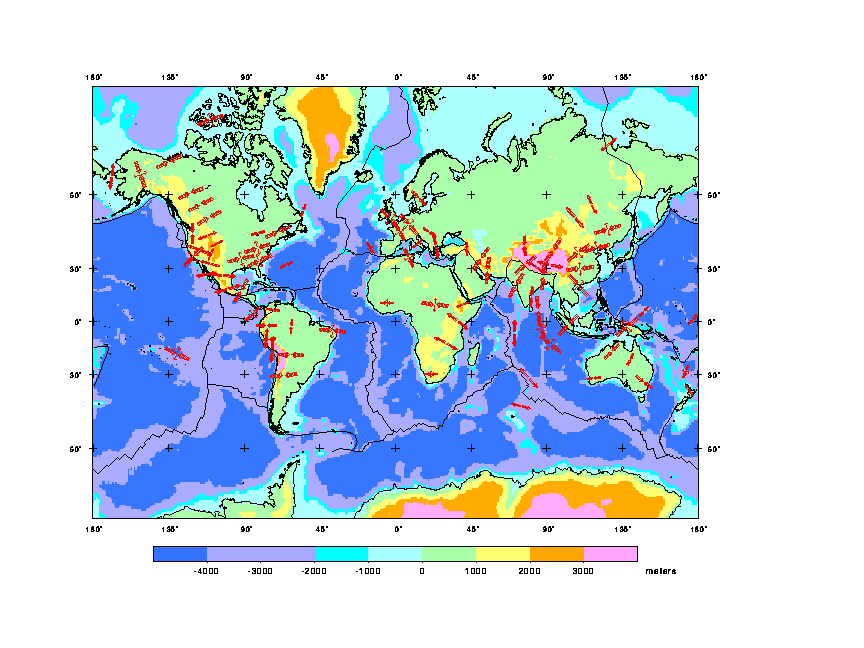
Figure 6 : World Stress Map Project's averaged maximum stress data (red
arrows). Color contour representative of elevation. After Zoback
et. al. (1992) .
Numerous observations suggest that drag forces and resisting forces do not strongly control the stress field of the uppermost brittle part of the lithosphere. The general state of compression in the old oceanic lithosphere (older than ~80 Ma) indicates that the integrated ridge push force dominates over the associated mantle drag forces (Richards et al., 1992). Also, the predicted stresses related to whole mantle flow inferred from seismic tomography do not match well with the broadest scale tectonic stress data, especially when compared to the correlation of the boundary and body forces with tectonic directions (Zoback, 1992).
Correlations between the World Stress Map's tectonic stress measurements
and PDF's were immediately obvious after the measurements were plotted
on a map of the Earth's plate boundaries. Normal faults that showed maximum
tension perpendicular to ridge crests were seen for most of the world's
spreading ridges. Old oceanic crust (>35 Ma) experiences mainly thrust
or strike-slip faulting. This tectonic style is consistent with an intra-plate
stress field dominated by compression associated with the net slab and/or
ridge forces. Orientations of compressional stresses which dominate the
interiors of most continental cratons, most importantly North America and
Western Europe, are similar to those predicted for ridge push and slab
forces (Zoback, 1992; Richards, 1992). Furthermore, stress measurements
show, on a broad scale, stress fields changing in style (i.e. compressional
to tensional) over individual plates with a tendency for the maximum horizontal
stress direction (Sh Max) to be parallel to the absolute plate
motion. This last fact is an important observation which directly relates
to the relationship between plate boundary and body forces and the motion
of plates. Using the evidence provided by the WSMP that plate boundary
and body forces appear to dominate the driving mechanism of plate motion,
the next step is to quantify the different magnitudes of the individual
PDF's.
![]() The
Techniques of Quantifying PDF'S
The
Techniques of Quantifying PDF'S
Before we begin to tackle the problem of quantifying PDF's, we must
understand the inherent difficulty in making inferences about plate driving
motions from kinematics. The difficulty lies in the physical equation that
states that the motion of a rigid plate is the integrated effect of all
summed individual torques acting on that plate. The magnitudes of these
individual torques, however, are non-unique and unconstrained. The simple
example below shows how different combinations of coefficients, or in this
case scalar magnitudes of torque, can lead to the same outcome.
Example:
xTA + yTB + zTC + wTD
= 0
1 + 3 + -2 + -2 = 0
2 + 2 + 2 + -6 = 0
1 + -1 + 1 + -1 = 0
Another difficulty with using analytical or numerical methods to account for the large group of PDF's is the complexity of the multiple plate boundary conditions and relations. Nevertheless, it has been shown that against an absolute reference frame we can come up with a relatively accurate solution (Forsyth and Uyeda, 1975; Carlson et al., 1983). By solving an inverse problem with a known absolute motion reference frame, we can estimate relative magnitudes for individual plate driving forces.
There are essentially three different techniques geologists and geophysists use in order to quantify the different plate driving forces. Deformational modeling studies using intra-plate stress fields were popular in the late 1970's and early 1980's. Some of the earlier attempts included Solomon et al. (1975), Richards et al. (1975), and Bott (1991). These studies used finite element models in an attempt to predict both global and single plate motions based on the forces driving and resisting the individual plates. The results of these models worked well for individual boundaries and even for some of the individual plates, but integrated over the entire globe, the model broke down and did not adequately account for all of the appropriate complex boundary conditions. A second approach was based on empirical relationships between plate size, age, type, geometry, motion, and velocity (Forsyth and Uyeda, 1975; Carlson et al., 1983). From these relationships strong correlations between plate velocities and the age of oceanic lithosphere were derived. However, this method did not allow for other types of forces other than those associated with the subducting slab, such as basal drag, tectonic resistance, etc.
The third approach, and the method I feel to be the most important and
informative, is the Net Torque Method. This technique studies the driving
mechanism of plate motion by balancing the net torque acting on each plate
(Forsyth and Uyeda, 1975; Chapple and Tullis, 1977). The advantage of this
method is the incorporation of all Plate Driving Forces into the equation,
both driving and resistive. Inherent is the important concept that the
net torque acting on a plate is ultimately responsible for a plate's motion.
The laws of rigid body rotation state that if there is no acceleration
and/or inertia acting on that body, then all applied forces, or torques,
must sum to zero. It follows that the net torque acting on that body must
also be zero, by definition. This is Newton's second law of motion which
states that the acceleration of any object is directly proportional to
the net force acting on it, and inversely proportional to its mass. This
property is central in determining the relative magnitudes of the torques
acting on an individual plate (fig. 7).
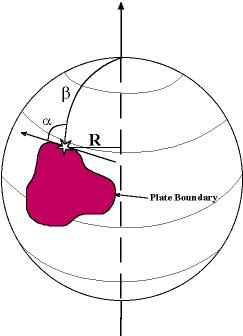 Figure
7 : Schematic diagram showing the different mathematical components of
a torque acting on a lithospheric plate. (R) is the radial distance from
the axis of rotation, or lever arm distance, (Beta) is the co-latitude
position of the plate boundary, and (alpha) is the angle between the strike
of the boundary and the azimuth to the pole of the torque axis. Figure
taken from Forsyth and Uyeda (1975).
Figure
7 : Schematic diagram showing the different mathematical components of
a torque acting on a lithospheric plate. (R) is the radial distance from
the axis of rotation, or lever arm distance, (Beta) is the co-latitude
position of the plate boundary, and (alpha) is the angle between the strike
of the boundary and the azimuth to the pole of the torque axis. Figure
taken from Forsyth and Uyeda (1975).There are several basic assumptions that must be made in order for the
Net Torque Model to work. It is assumed that the inertia and acceleration
of the individual plates are nonexistent or negligible, and thus the
plates are in dynamic equilibrium. The boundary and body forces,
for this problem, are considered the main driving forces as opposed to
active-mantle flow. And lastly, because the plates are confined to move
on the surface of the globe, their respective motions are, by definition,
described as a rotation about an axis passing through the center of the
Earth. If, as assumed, there is no acceleration, the sum of the net torques
will add to zero.
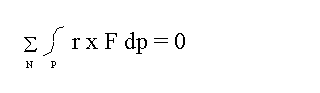 Equation
1: Basic equation showing that the product of the lever arm distance, or
radial distance from center of rotation, and the applied force equals zero
when summed over a plate of area (P) .
Equation
1: Basic equation showing that the product of the lever arm distance, or
radial distance from center of rotation, and the applied force equals zero
when summed over a plate of area (P) .With these assumptions we can then determine the relative magnitudes
of the forces that minimize the net torque acting on each plate. The inverse
problem that determines the relative strengths of each of the different
PDF's is solved with respect to an inferred absolute reference frame. Today
the world's hot spots are our best source for an absolute plate motion
reference frame. In this case we are assuming the mantle is fixed with
respect to the Earth's axis of rotation. To solve the inverse problem a
matrix must be created with the known number of forces and plates and the
unknown scalars for the different forces. The basic equation is then solved
for each plate in three dimensions as follows:
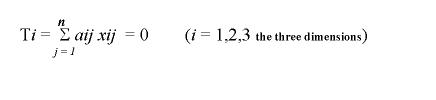 Equation
2 : Variables are listed below.
Equation
2 : Variables are listed below.where n is the number of forces acting on the plate, xij is the coefficient of magnitude (scalar) of the jth force, and aij holds all the physical and geometrical constants of the plate. If there is a real solution, the determinant must equal zero. Once the possibility of a real solution is found, the next step is to solve for the unknowns (xij ) by a least squares method. Since we know the solution to the scaled matrix is zero, a least squares method can be used to retrieve n eigenvectors for which several eigenvalues can be found. Here is where a problem arises. As a consequence to the existence of several solutions, or eigenvalues for each scalar (xij) , the coefficients become non-unique. However, the degree to which the solution is non-unique can be estimated and minimized.
The non-unique solution may at first glance be perceived as a huge drawback, but the relative magnitude of the forces involved are found with accuracy. In two of the most referenced torque studies, Forsyth and Uyeda (1975) and Tullis and Chapple (1973), the pulling of the slab and the collisional resistance from the mantle provide the dominant role in controlling plate motion. The remaining forces for these two models have the same relative magnitude, and thus can not be uniquely determined. A more detailed analysis of the least squared no net torque method can be found in Forsyth and Uyeda (1975) and Tullis and Chapple (1973).
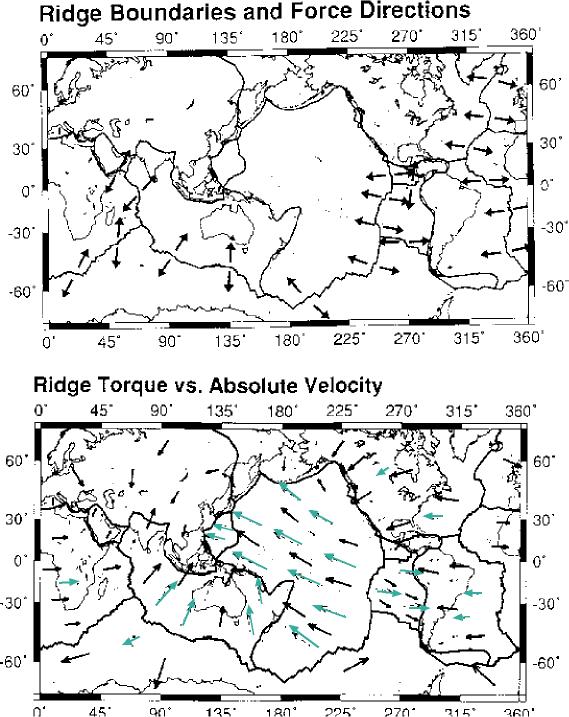
Richards (1992) did a detailed Net Torque analysis combined with data
from the World Stress Map Project to better understand and resolve the
remaining force magnitudes (fig. 8). He found that the ridge push force
exhibits a strong correlation with the azimuth of the absolute velocity
of the plates. This correlation suggests an alternative explanation for
the alignment of intra-plate stresses and absolute plate motion. The relationship
between ridge push forces on intra-plate stresses is also consistent with
slab forces being an important component of the plate driving mechanism.
Because of the equal and opposite nature of the slab pull and collisional
resistant forces, the sum net slab force contributes relatively little
to the deformation, or stress field, of the surface plates. Therefore other
forces must account for our observations, namely ridge push.
To the question, "What drives plate tectonics?" we have presented two options: (1) mantle convection, and (2) lithospheric plate boundary and body forces. It is in the opinion of this author that it is the plates themselves that are the dominant source of force involved in the absolute movement of the lithospheric plates over the surface of the Earth. The strong correlations between observed tectonic stress and absolute plate motions shown by the World Stress Map Project point directly to the present lithospheric stress fields being dominated by the individual plate boundary and body forces (Zoback et al., 1989, Zoback, 1992). These observations, along with the Net Torque Model, allow us to begin to put a coherent story together in terms of the relative magnitudes of different PDF's. Although the slab forces (slab pull and collisional resistance) dominate the other PDF's, their equal and opposite nature allows ridge push to be the most important observable plate driving force.
This solution for Plate Driving Forces works for today, but what about the past? Did slab and ridge forces always dominate, and did they always dominate in that order? These questions are important when considering the driving forces behind plate motion over time. Plates must rearrange themselves throughout supercontinent cycles, continuously changing the constructive and destructive nature of their boundaries. It is logical to assume then that these changes in the interactions and movements of plates must also change the relative importance of different PDF's in time and space. It follows then that the forces that drive plates are depenent on the nature of boundary conditions and plate arrangement through time.
There are still many unanswered questions related to PDF's. Can plate
driving forces be responsible for the breakup of supercontinents? Are plate
boundary and/or plate body forces responsible for the initiation of subduction
zones and spreading ridges? Most researchers believe in these special cases,
mantle forces related to large convection cells must dominate the driving
forces (Jacoby, 1980; Carlson et al, 1983;Wilson, 1991; Zeigler, 1991).
So, in a sense, it is because of the present condition that we have today's
magnitudes and effective forces, and through time the dominant forces will
change from plate to mantle and back. After all, is it not the mantle itself
that inevitably supplies the energy and heat that runs the system?
"Plates could not move, or even exist, if not for the Earth's heat which must be removed from its interior to the surface through mantle convection. In this sense the mantle drives the plates, for it is the interior of the Earth that is the ultimate source of energy of all motion."
(Runcorn, 1980)
![]()
Bott, M.H.P., 1993. Modeling the Plate-Driving Mechanism, Journal
of the Geological Society, 150: p 941-951.
Bott, M.H.P, 1991. Ridge Push and Associated Plate Interior Stress in
Normal and Hot Spot Regions, Tectonophyics, 200: p 17-32.
Bott, M.H.P., 1991. Sublithospheric Loading and Plate-Boundary Forces.
In: Whitmarsh, R.B., Bott, M.H.P., Fairhead, J.B. & Kusznir, N.J.
(eds) Tectonic Stress in the Lithosphere. Philosophical Transactions
of the Royal Society, London, p 83-93.
Carlson, R.L., 1983. Plate Motions, Boundary Forces, and Horizontal
Temperature Gradients:Implications for the Driving Mechanism, Tectonophysics,
99: 149-164.
Carlson, R.L, et al., 1983. The Driving Mechanism of Plate Tectonics
: Relation to Age of the Lithosphere at Trenches, Geophysical Research
Letter, 10: p 297-300.
Chapple, W.M., and Tullis, T.E., 1977. Evaluation of the Forces that
Drive the Plates, Journal of Geophysical Research, 82: p 1967-1984.
Doglioni, C., 1990. The Global Tectonic Pattern. Journal of Geodynamics,
12: p 21-38.
Fleitout, L, 1991. The Sources of Lithosperica Tectonic Stresses. In:
Whitmarsh, R.B., Bott, M.H.P., Fairhead, J.B. & Kusznir, N.J. (eds)
Tectonic Stress in the Lithosphere. Philosophical Transactions of the
Royal Society, London, p 73-81.
Hager, B.H., and O'Connell, R.J., 1978. Subduction Zone Dip Angles and
Flow Driven by Plate Motion, Tectonophysics, 50: p 111-133.
Jacoby, W.R., 1980. Plate Sliding and Sinking in Mantle Convection and the Driving Mechaism, In:
Davis, P.A., Runcorn, F.R.S. (eds) Mechanisms of Continental Drift and Plate Tectonics,
Academic Press : p 159-172.
Jurdy, D.M., and Stefanick, M., 1991. The Forces Driving the Plates:
Constraints from Kinematics and Stress Observations. In: Whitmarsh,
R.B., Bott, M.H.P., Fairhead, J.B. & Kusznir, N.J. (eds) Tectonic
Stress in the Lithosphere. Philosophical Transactions of the Royal Society,
London, p 127-139.
McKenzie, D.P., 1969. Speculations on the Consequences and Causes of
Plate Motions, The Geophysical Journal, 18: 1-32.
McKenzie, D.P., 1968. The Influence of the Boundary Conditions and Rotation
on Convection in the Earth's Mantle, The Geophysical Journal, 15:
457-500
Middleton, G.V., Wilcock, P.R., 1996. Mechanics in the Earth and Environmental Sciences. Cambridge
University Press, Australia : pp 496.
Pavoni, N., 1993. Pattern of Mantle Convection and Pangaea Break-up,
as revealed by the evolution of the African plate, Journal of Geological
Society, 150: p 953-964.
Richardson, R.M., 1992. Ridge Forces, Absolute Plate Motions, and the
Intraplate Stress Field, Journal of Geophysical Research, 97: 11,739-11,748.
Runcorn, S.K., 1980. Some Comments on the Mechanism of Continental Drift, In: Davis, P.A., Runcorn,
F.R.S. (eds) Mechanisms of Continental Drift and Plate Tectonics,
Academic Press : p 193-198.
Vigny, C., et al., 1991. The Driving Mechanism of Plate Tectonics,
Tectonophysics, 187: p 345-360.
Wilson, M., 1993. Plate-moving Mechanisms: Constraints and Controversies,
Journal of the Geological Society, 150: p 923-926.
Wilson, M., 1993. Geochemical Signatures of Oceanic and Continental
Basalts: A Key to Mantle Dynamics, Journal of Geological Society,
150: p 977-990.
Wortel, M.J.R, et al., 1991. Dynamics of the Lithosphere and
the Intraplate Stress Field. In: Whitmarsh, R.B., Bott, M.H.P.,
Fairhead, J.B. & Kusznir, N.J. (eds) Tectonic Stress in the Lithosphere.
Philosophical Transactions of the Royal Society, London, p 111-126.
Wortel, M.J.R., and Vlaar, N.J., 1976. Lithospheric Aging, Instability and Subduction, Tectonophysics,
32: p 331-351.
Ziegler, P.A., 1992. Plate Tectonics, Plate Moving Mechanisms and Rifting,
Tectonophysics, 215: p 9-34.
Ziegler, P.A., 1993. Plate-moving Mechanisms: their Relative Importance,
Journal of Geological Society, 150: p 927-940
Zoback, M.L., 1992. First and Second Order Patterns of Stress in the
Lithosphere: The World Stress Map Project, Journal of Geophysical Research,
97: p 11,703-11,728.
Zoback, M.L., and Magee, M., 1991. Stress Magnitudes in the Crust: Constraints
from Stress Orientation and Relative Magnitude Data. In: Whitmarsh,
R.B., Bott, M.H.P., Fairhead, J.B. & Kusznir, N.J. (eds) Tectonic
Stress in the Lithosphere. Philosophical Transactions of the Royal Society,
London, p 181-194.
Zoback, M.L., et al., 1989. Global Patterns of Tectonic Stress,
Nature, 341: p 291-298.